Correct Answer is Option (B)
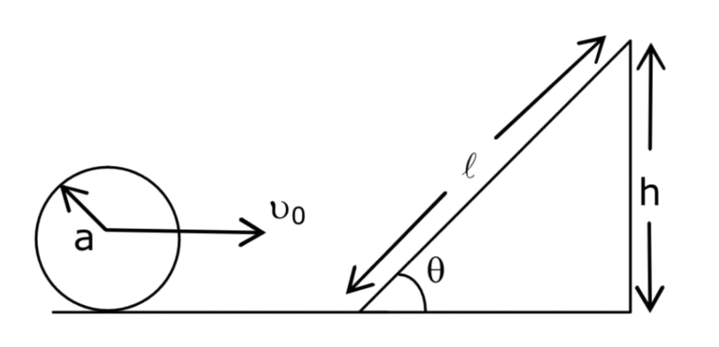
From energy conservation
mgh =
mgh =
gh =
gh =
h =
from
triangle,
then h =
l sin
21. (JEE Main 2021 (Online) 25th February Evening Shift)
A sphere of radius 'a' and mass 'm' rolls along a horizontal plane with
constant speed v0. It
encounters an inclined plane at angle and climbs upward. Assuming
that it rolls without
slipping, how far up the sphere will travel?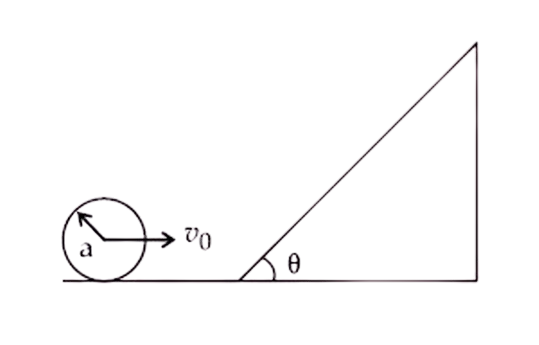
A.
B.
C.
D.
Correct Answer is Option (B)

From energy conservation
mgh =
mgh =
gh =
gh =
h =
from
triangle,
then h =
l sin
22. (JEE Main 2021 (Online) 22th July Evening Shift )
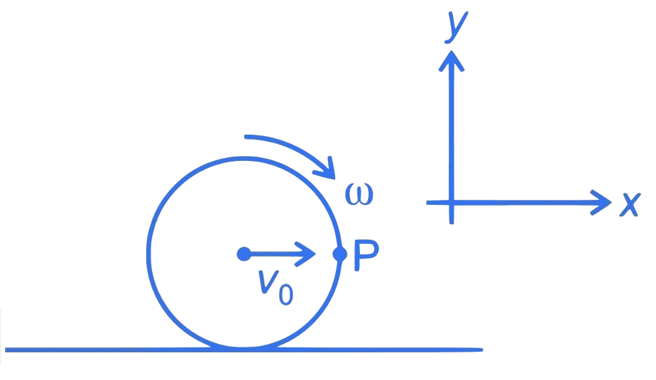
The centre of a wheel rolling on a plane surface moves with a speed v0. A particle on the rim of the wheel at the same level as the centre will be moving at a speed . Then the value of x is _____________.
Correct answer is (02)
23. (JEE Main 2021 (Online) 20th July Evening Shift )
Two bodies, a ring and a solid cylinder of same material are rolling down without slipping an inclined plane. The radii of the bodies are same. The ratio of velocity of the centre of mass at the bottom of the inclined plane of the ring to that of the cylinder is . Then, the value of x is _____________.
Correct answer is (3)
I in both cases is
about point of contact
Ring
mgh =
mgh =
Solid
cylinder
mgh =
mgh
24. (JEE Main 2021 (Online) 20th July Morning Shift )
A circular disc reaches from top to bottom of an inclined plane of length 'L'. When it slips down the plane, it makes time 't1'. When it rolls down the plane, it takes time t2. The value of is . The value of x will be _______________.
Correct answer is (2)
According to
question, a circular disc reaches from top to
bottom of an inclined plane of length L. This can be
shown as

When the disc slips down the inclined plane, it
takes time t1. Therefore, in this
case its acceleration, a1 = g sin
s =
ut1 + a1t
s = g sint ....
(i)
And when the disc rolls down
the inclined plane, it takes time t2.
Therefore in this case, its
acceleration,
[ for
disc, ]
s =
ut2 +
a2t = . g sin t ....
(ii)
On dividing Eq. (i) by Eq.
(ii), we get
....
(iii)
According to question,
value of is .
Comparing
it with Eq. (iii), we get
x = 2
25. (JEE Main 2021 (Online) 17th March Morning Shift )
The following bodies,
(1) a ring
(2) a
disc
(3) a solid cylinder
(4) a solid
sphere,
of same mass 'm' and radius 'R' are
allowed to roll down without slipping simultaneously from
the top of the inclined plane. The body which will
reach first at the bottom of the inclined plane is
___________. [Mark the body as per their respective
numbering given in the question]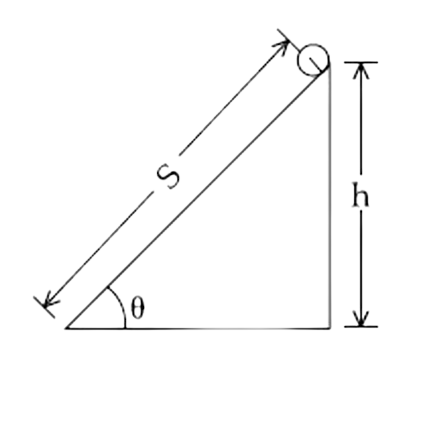
Correct answer is (4)
IR
= mR2,
aR = g sin/2
ID
= ,
aD = g
sin
ISC
= ,
aSC = g
sin
ISC
= mR2,
aSS =
g
sin
S
= ut + at2,
t
=
t
solid
sphere will take minimum time.