Correct Answer is Option (B)
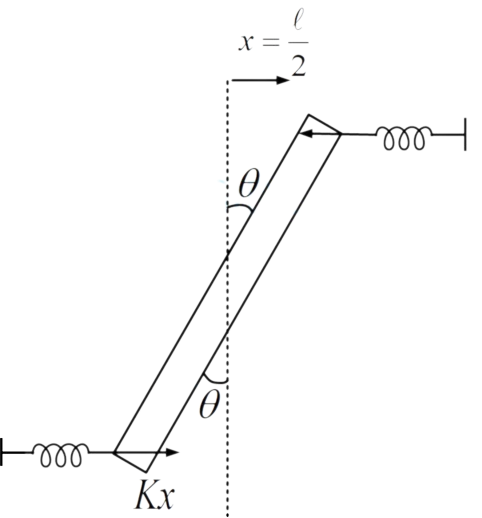
1.(JEE Main 2019 (Online) 12th January Morning Slot)
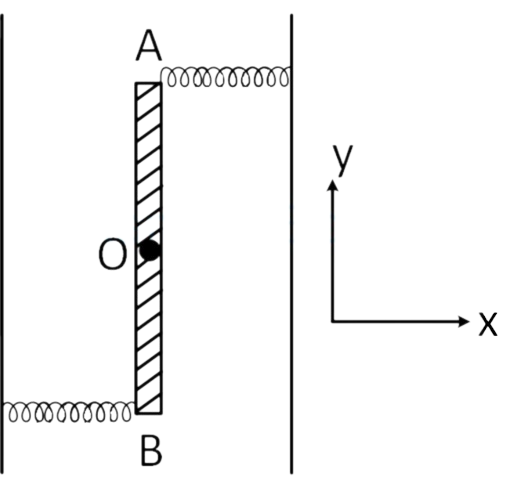
Two light identical springs of spring constant k are attached horizontally at the two ends of a uniform
horizontal rod AB of length and mass m. The rod is pivoted at its centre 'O' and
can rotate freely in horizontal plane. The other ends of the two springs are fixed to rigid supports as
shown in figure. The rod is gently pushed through a small angle and released. The frequency of resulting
oscillation is :
A.
B.
C.
D.
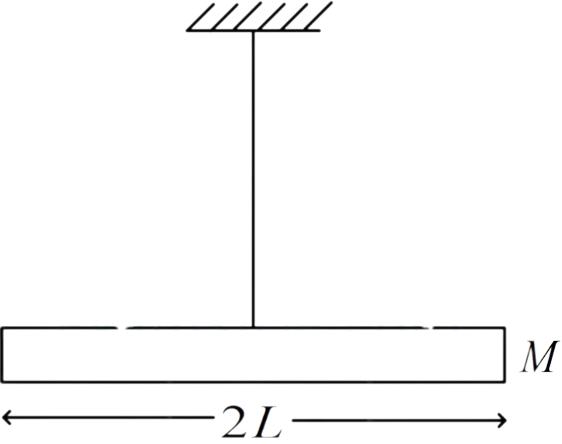
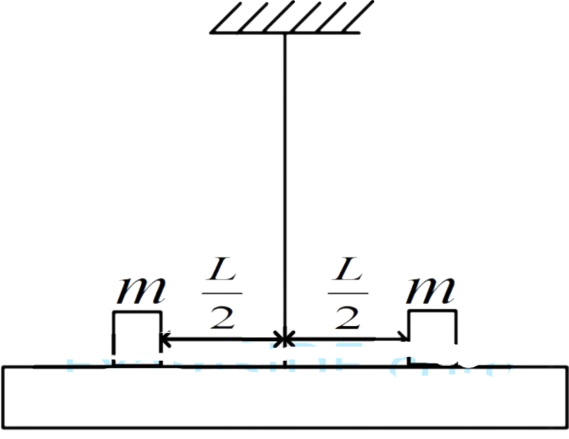
2. (JEE Main 2019 (Online) 9th January Evening Slot)
A rod of mass 'M' and length '2L' is suspended at its middle by a wire. It exhibits torsional oscillations; If two masses each of 'm' are attached at distance 'L/2' from its centre on both sides, it reduces the oscillation frequency by 20%. The value of radio m/M is close to :
A. 0.77
B. 0.57
C. 0.37
D. 0.17
Correct Answer is Option (C)
Initially :
After putting 2 masses of each 'm' at a distance from center :
We know,
Time period (T) = 2
T
Frequency (f)
=
Also given that,
After putting two masses 'm' at both end new frequency becomes 80% of initial frequency.
f2 =
0.8f1
=
= 0.64
Initial moment of inertia of the system,
=
Final moment of inertia of the system,
I2 = + 2
= 0.64
= +
=
= = 0.37